The orbital period of two stars is given by the equation,
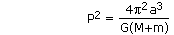
where
P is the period,
a is the semimajor axis (the distance between the stars),
G is the constant of gravitation, and
M and
m are the masses of the two stars.
To get the period of C, I suppose you would just treat AB as a single mass located at the barycenter, with the semimajor axis being the distance between C and the AB barycenter. Proceed similarly for the ABC and DE period.
In such a large system as this the periods would undoubtedly be effected by perturbations from the different stars, but in your case I'd ignore that. Calculating each period as a simple two-body problem would probably be close enough.